Right triangle trigonometry
The entirety of trigonometry is based on similarity and right triangles.
Contents
Trigonometric ratios

For an acute angle in a right triangle, we define
as ratios of side lengths in a right triangle.
For similar triangles, , , and remain the same, despite changes in side lengths. In other words, the trigonometric ratios only depend on the angle, so they are also called trigonometric functions.
In right triangles, trig ratios are useful when you need to find a missing side.
To find the angle, use the , , and functions of the ratio of the sides.
Example: Using a diagram or otherwise, simplify
From the Pythagorean identity, we have
which corresponds to a right triangle with and legs , , and hypotenuse . can be interpreted as the base of a larger triangle while keeping the height of . Here is a diagram.

From sum of angles in triangle , we have
From supplementary angles and sum of angles in triangle , we have
Since , triangle is isosceles, meaning the two remaining angles are equal and must be . Here is a diagram with all the new information as well.

Then by definition of , the desired angle is with opposite side and adjacent .
Inverse trigonometric functions
Inverse trig functions , , return the angle when you have the ratio . They are useful for missing angles.
Applications
definitions
The angle of elevation is the angle from horizontal up to some point of interest. The angle of depression is the angle from horizontal down to some point of interest.
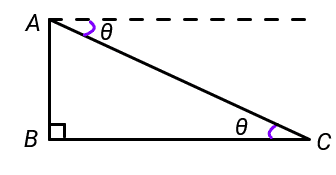
right triangles in 3-D
Look to identity right triangles in three dimensions. If the two sides to an angle span different basis directions, then they meet at right angles. For example if spans only the and directions, while spans only the direction, then is a right angle.
strategies
- Draw a diagram, label important points and given angles and sides (distances).
- Light travels in a straight line.
- Identify right angles.
- Identify similar triangles.
- Reuse variables when possible. For example if total distance is , you can label part of it and the remaining .
- Verify your calculator is in degrees mode, when working with angles in degrees.
See also
For exact trigonometric ratios that you should remember, see Exact ratios